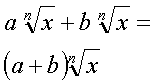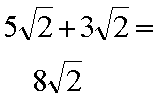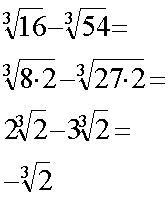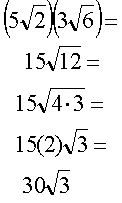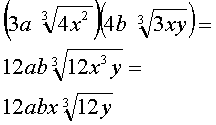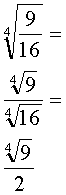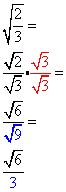|
 |
|
|
|
||
|
Operations with Radicals |
||
|
Simplification Addition and Subtraction Multiplication Division
|
||
| Simplification of Radicals | Rule | Example | |
|
Use the two laws of radicals to
SHORTCUT:
|
|
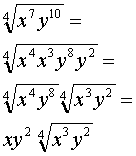
|
|
| Top | |||
|
Simplification Addition and Subtraction Multiplication Division |
|||
|
|
|||
| Addition and Subtraction of radicals | Rule | Example | |
|
Simplify all radicals. Combine only
like radical terms: Combine like radicals by combining the coefficients of the radical terms. The coefficient is the factor that sits outside the radical to the left. |
Note:
|
Example 1:
Example 2:
|
|
| Top | |||
|
Simplification Addition and Subtraction Multiplication Division |
|||
|
|
|||
| Multiplication of radicals | Rule | Example | |
|
If the indices are the same
NOTE: You may simplify the radicals before multiplying. However, you may need to simplify the radical again once you have found the product.
|
Example 1:
Example 2:
|
||
| Top | |||
|
Simplification Addition and Subtraction Multiplication Division |
|||
|
|
|||
| Division | Rule | Example | |
| NOTE: A simplified radical contains no fractions and no radicals in the denominator. | |||
Division
Type I:
|

|
|
|
|
Division
Type II: |
|
|
|
(a + b) and (a - b) are conjugates: middle signs are opposite. Multiplying
by conjugates gives the difference of squares:
|
|
|
|
| Top | |||
|
Simplification Addition and Subtraction Multiplication Division |
|||
|
|
|||